Scale drawings: word problems
Key notes :-
1. What are Scale Drawings?
- A scale drawing is a drawing that represents an object or a place with all its parts in proportion to the actual size.
- The scale tells you how the drawing relates to the real object. For example, a scale of 1 cm: 5 m means that 1 cm in the drawing represents 5 meters in real life.
2. Understanding the Scale
- The scale is written as a ratio or a fraction (e.g., 1:100, 1/100, or 1 cm: 1 m).
- Numerical Representation:
- 1:10 scale means every 1 unit on the drawing represents 10 units in reality.
- 1 cm: 10 m means 1 cm in the drawing equals 10 meters in real life.
3. How to Solve Word Problems
- Identify the Scale: Find the scale given in the problem (e.g., 1 cm: 5 km).
- Interpret the Question: Read carefully to understand what the problem is asking (e.g., finding the actual length of a building when given its drawing).
- Set Up a Proportion: Use the scale to set up a proportion, where you compare the measurements in the drawing with the actual measurements.
4. Example of Word Problem
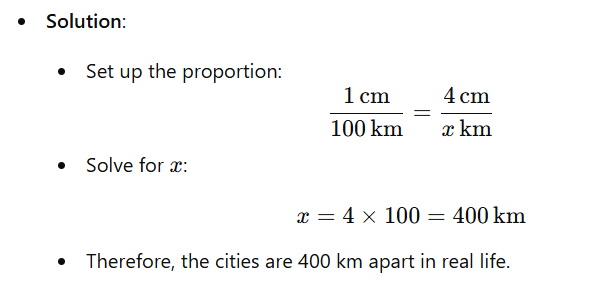
- Problem: A map has a scale of 1 cm: 100 km. If the distance between two cities on the map is 4 cm, how far apart are the cities in real life?
Learn with an example
🔥After a long hike in a national park, Joe decides to go relax at the beach. The parking permit he purchased allows him to park at any state beach without paying again. While consulting a map, Joe notices that the closest state beach is 9 centimetres away from the park. The scale of the map is 1 centimetre = 1 kilometre. In real life, what is the distance between the national park and the closest state beach?
______ kilometres
- Write a ratio that represents the scale of the map in centimetres to kilometres: 1/1,
- Write a ratio that relates the centimetres between the state park and the closest state beach on the map to the kilometres between the state park and the closest state beach in real life: 9/d,
- Use those ratios to set up a proportion and solve.
- 1/1 = 9/d
- 1/1 ( d ) = 9/d ( d ) ——-> Multiply both sides by d
- d = 9 ——-> Simplify
- In real life, the distance between the national park and the closest state beach is 9 kilometres.
🔥Judy is on a road trip and notices that, according to the map, the next petrol station on her route is 7 centimetres away. If the scale of the map is 1 centimetre = 10 kilometres, then what is the actual distance between the two petrol stations?
______ kilometres
- Write a ratio that represents the scale of the map in centimetres to kilometres: 1/10.
- Write a ratio that relates the centimetres between the two petrol stations on the map to the kilometres between the two petrol stations in real life: 7/d.
- Use those ratios to set up a proportion and solve.
- 1/10 = 7/d
- 1/10 ( 10d ) = 7/d ( 10d ) ——-> Multiply both sides by 10d
- d = 10 · 7 ——–> Simplify
- d = 70 ———> Simplify
- The actual distance between the two petrol stations is 70 kilometres.
🔥On a public transportation map, two stations are 6 centimetres apart, whereas in real life the distance between them is 2 kilometres. What is the map’s scale?
Write your answer in the simplest form using whole numbers.
______ centimetres = _______ kilometres
- Write a ratio that relates the centimetres between the two stations on the map to the kilometres between the two stations in real life: 6/2.
- To find the scale of the map, rewrite this ratio in simplest form using whole numbers.
- 6/2 = 3/1 —–> Divide the numerator and denominator by the GCF, which is 2
- The map’s scale is 3 centimetres = 1 kilometre.
Let’s practice! 🖊️

