Understanding ratios
Key points :-
1. What is a Ratio?
- A ratio is a way to compare two quantities.
- It tells you how much of one thing there is compared to another.
- The ratio of two numbers can be written in three forms:
- A to B (e.g., 3:4)
- A/B (e.g., 3/4)
- A is to B (e.g., 3 is to 4)
2. Simplifying Ratios:
- Like fractions, ratios can be simplified by dividing both parts of the ratio by their greatest common divisor (GCD).
- Example: The ratio 6:8 can be simplified to 3:4 by dividing both 6 and 8 by 2.
3. Equivalent Ratios:
- Two ratios are equivalent if they simplify to the same ratio.
- Example: 4:6 is equivalent to 2:3 because both can be simplified by dividing by 2.
4. Ratio as a Fraction:
- A ratio can be expressed as a fraction.
- Example: 3:5 can be written as 3/5.
- This helps in finding proportions and solving problems involving ratios.
5. Part-to-Part and Part-to-Whole Ratios:
- Part-to-Part: Compares one part of a whole to another part. Example: The ratio of boys to girls in a class is 3:2.
- Part-to-Whole: Compares one part of a whole to the entire whole. Example: The ratio of boys to the total class is 3:5.
Learn with an example
✈️ What is the ratio of rectangles to total shapes?

✈️ Write your answer as a fraction. Use a forward slash ( / ) to separate the numerator and denominator. _____
- Count the number of rectangles. There is 1 rectangle.
- Count the total number of shapes. There are 2 shapes.
- Write the ratio of rectangles to total shapes: 1/2
✈️ What is the ratio of stars to hexagons?

- Count the number of stars. There are 5 stars.
- Count the number of hexagons. There are 3 hexagons.
- Write the ratio of stars to hexagons: 5/3
✈️ What is the ratio of hexagons to circles?
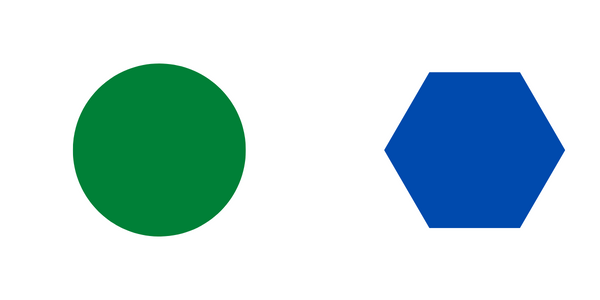
✈️ Write your answer as two numbers separated by a colon (for example, 2:3).
- Count the number of hexagons. There is 1 hexagon.
- Count the number of circles. There is 1 circle.
- Write the ratio of hexagons to circles: 1:1
Let’s practice! 🖊️

