Convert between standard and scientific notation
key notes :
🏹 Scientific notation:
You can use scientific notation to write very large or very small numbers. Here are some examples.
4,000,000 in scientific notation is 4×106.
0.00007 in scientific notation is 7×10–5.
Writing numbers in scientific notation can make them easier to work with.
How do you read numbers in scientific notation?
You can interpret scientific notation by converting the number to standard form. Just follow the rules for multiplying by a power of 10. When you multiply by a power of 10, the exponent in the power of 10 tells you how many places to move the decimal point. Follow these rules:
- If the exponent is positive, move the decimal point to the right.
- If the exponent is negative, move the decimal point to the left.
Let’s try it! The planet Neptune is about 2.78×10⁹ miles from the sun. Write 2.78×10⁹ in standard form.
The exponent in 10⁹ is positive 9, so move the decimal point 9 places to the right.
So, 2.78×10⁹ in standard form is 2,780,000,000.
Another example:
The average grain of sand weighs about 4.4×10⁻⁶ kilograms. Write 4.4×10–6 in standard form.
The exponent in 10⁻⁶ is negative 6, so move the decimal point 6 places to the left.
So, 4.4×10⁻⁶ in standard form is 0.0000044.
How do you write numbers in scientific notation?
You can write numbers in scientific notation by writing them as a product of two factors.
- The first factor is always a number greater than or equal to 1 but less than 10.
- The second factor is always a power of 10.
For example, you can write the number 561,000,000 in scientific notation as 5.61×10⁸.
To determine the two factors, follow these steps:
- Move the decimal point in the number until you get a number greater than or equal to 1 but less than 10. This will be the first factor.
- Count how many places, and in which direction, you moved the decimal point. This will tell you the exponent in the power of 10. If you moved the decimal point to the left, your exponent will be positive. If you moved the decimal point to the right, your exponent will be negative.
🏹 Let’s try it! Write 0.000095 in scientific notation.
Start by writing the first factor. Move the decimal point between 9 and 5. The first factor is 9.5.
Then, determine the exponent in the power of 10. Count the number of places the decimal point moved to make 9.5. Since you’ll need to move the decimal to the right, use a negative exponent.
The decim10⁻⁵.
So, the number 0.000095 in scientific notation is 9.5×10⁻⁵.
Learn with an example
How do you write 30 in scientific notation?
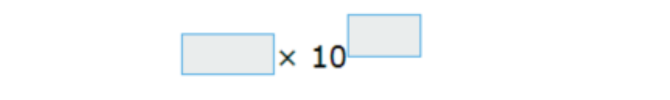
Move the decimal point to the left until the number is between 1 and 10. Count how many places you move the decimal point.
30 → 3.0
You moved the decimal point 1 place to the left. The power of 10 is 101.
30 = 3.0 × 101
How do you write 623 in scientific notation?
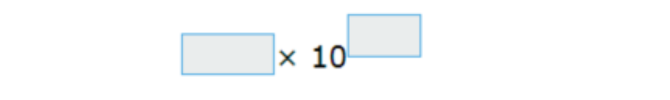
Move the decimal point to the left until the number is between 1 and 10. Count how many places you move the decimal point.
623 → 6.23
You moved the decimal point 2 places to the left. The power of 10 is 102.
623 = 6.23 × 102
How do you write 67,000 in scientific notation?
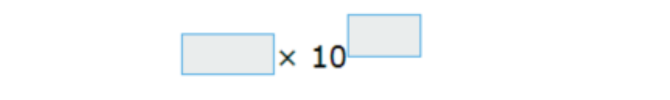
Move the decimal point to the left until the number is between 1 and 10. Count how many places you move the decimal point.
67,000 → 6.70
You moved the decimal point 4 places to the left. The power of 10 is 104.
67,000 = 6.70 × 104
Let’s practice!

